The pressure block design is designed to absorb forces caused by changes in the direction of liquid flowing through the tubes. A change in momentum creates external forces on the tubes. Pressure blocks are designed to accommodate these forces even though they cannot be supported by the pipe itself.
This article discusses the literature on pillar design and an example of pillar design.
CRIA Report 128 Provides comprehensive guidelines for column block design.
The calculation method is subject to the following assumptions, as indicated in the CRIA Report 128.
- The flow cross-sections are constant (except for cones).
- Viscosity loss in components is negligible, except for partially closed valves.
- The dynamic pressure is low (flow velocities rarely exceed 3 ms -1 which corresponds to a dynamic head < 0.05 bar).
- The theory of one-dimensional flow is valid
- The liquid in the tube is a Newtonian fluid, for example water.
- The thrust forces of any friction resulting from a pressure drop associated with a change in flow velocity are considered negligible for applications within the scope of this report.
Two methods are specified in the guideline to calculate thrust force.


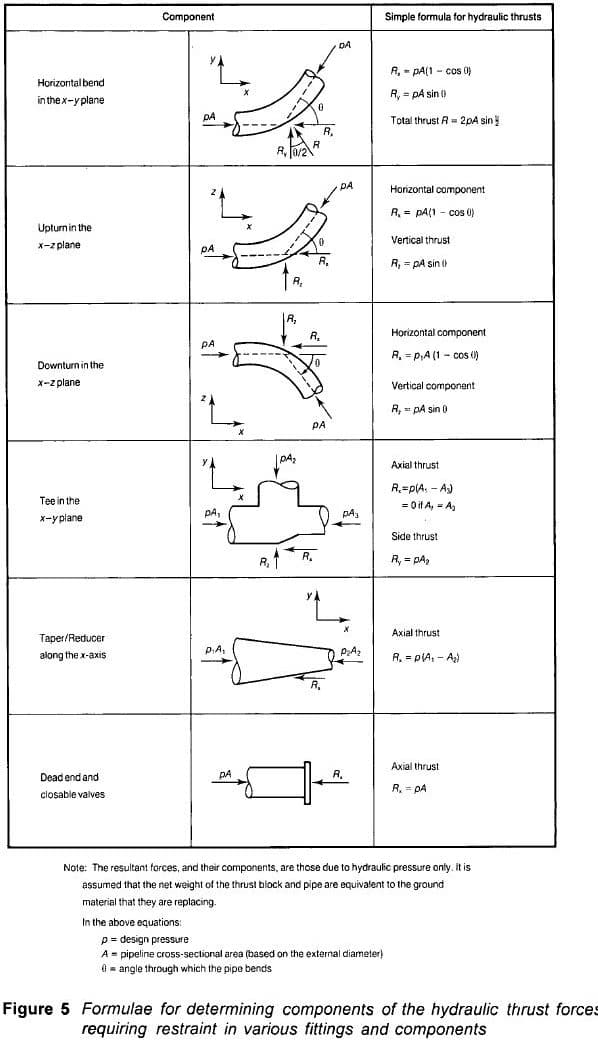
The following table and associated diagrams can also be used to calculate the forces acting on the column block.


To calculate the forces acting on the pressure block, you can use one of the methods mentioned above.
Example of pressure block construction
The analysis and design considered the following assumptions and design data.
- Concrete density 24 kN/m 3
- Class 25 concrete quality
- Minimum characteristic strength of steel 460 N/mm 2
- Reinforcement cover below the top edge of the soil 75mm
- Operating pressure 6bar (= 600kN/m 2 )
- Pipe inclination 45 0
- Internal friction angle 30 0
- Allowable support pressure 150 kN/m 2
- Soil density 18 kN/m 3
In this section, the calculation of the loads on the backrest block, the calculation of the weight of the backrest block and the verification of the support and reinforcement structure are carried out.
CALCULATION OF THE RESPONSE OF A 1200 MM DIAMETER TUBE
Pipe angle relative to the horizontal = 45 0
Horizontal force = PA(1-cosθ) = 600 x π x 1.2 2 (1-cos45)/4 = 200kN
The horizontal force acts in the direction of the tube.
Vertical response = PA sinθ = 600 x π x 1.2 2 x (sin45)/4 = 480 kN


Consider the arrangement of the thrust block as shown in the figure above. The width of the pressure block is 2250 mm and the length is 2000 mm.
Weight of concrete = (2.25 x 1.05 x 2 – π x 1.2 2 x 2 / (2 x 4) ) x 24 = 87 kN
Check the warehouse
Vertical reaction = 480 kN
Since operating pressure is taken into account when calculating thrust forces, the overlying vertical reaction can be taken into account to control the bearing.
Vertical force SLS = 480 kN
Vertical reaction to the ground = 480 + 87 = 567 kN
Pillar area = 2.25 x 2 = 4.5 m 2
Pressure under the base = 567 / 4.5 = 126 kN/m 2
The pressure is lower than the permitted bearing pressure (150 kN/m 2 ). So, okay.
Checking lateral stability
The change in angle creates vertical and horizontal forces. Horizontal forces act in the direction of the pipe. This calculation assumes that there are no forces acting on the pipe.


As shown in the figure above, the pipe is placed 2 m below the ground surface.
From the above calculation
Horizontal force on the tube (SLS) = 200 kN
Design force = 1.5 x 200 = 300 kN
Soil resistance = force due to difference in lateral force + base resistance
Grounding resistance = (σ P -σ A )A F +τ b A b
Calculate the active earth pressure coefficient = (1-sinθ)/(1+sinθ)
Ka = (1-sin30)/(1+sin30)
Ka = 0.333
Calculate the passive earth pressure coefficient = (1+sinθ)/(1-sinθ)
Kp = (1+sinθ)/(1-sinθ)
Kp = 1.5
Since the forces in the concrete block and tube, consider the pressure in the middle of the tube.
Active pressure = K A γh = 0.333 x 18 x 2 = 12 kN/m 2
Passive pressure = K P γh =3 x 18 x 2 = 108 kN/m 2
Side surface = tube + pillar = 0.6 + 1.05 = 1.65 m
Lateral area = 1.65 x 2 = 3.3 m 2
Resistance force = (108 – 12) x 3.3 = 316.8 kN
Applied horizontal force = 300 kN
Therefore, the pillar block is horizontally stable. Furthermore, the base resistance was not considered in this calculation. Taking into account the strength of the base increases the strength even further.
Furthermore, the above calculation can be verified as follows.
Applied force SLS = 200 kN
Resistance = 316.8kN
The safety factor against slipping = 316.8/200 = 1.58 >1.5
So, okay.