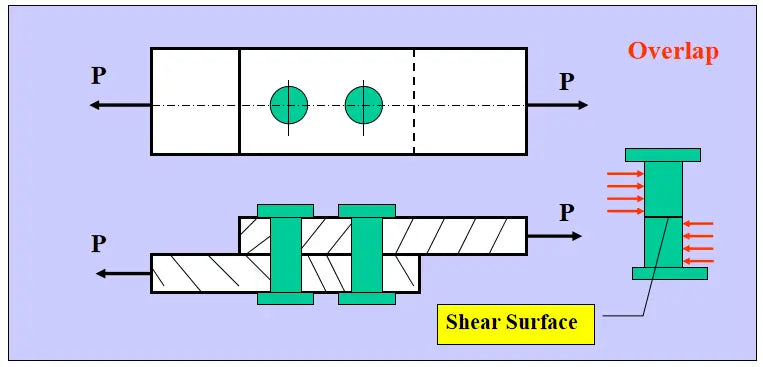
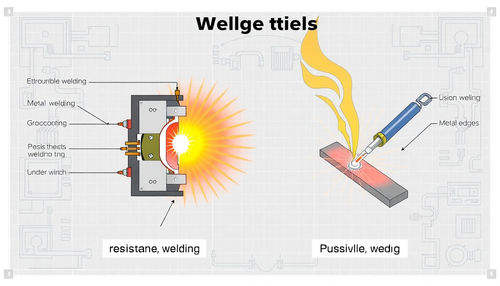
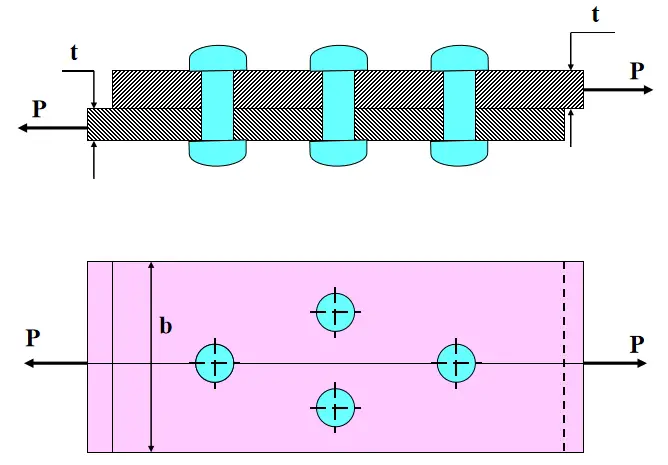
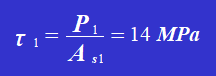Main types of rivet connections
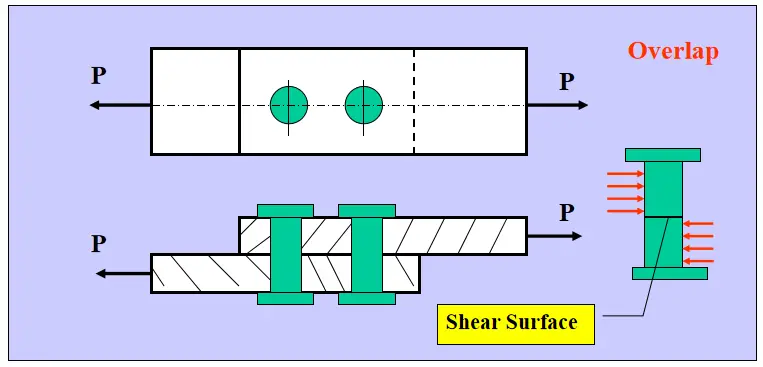
Single cover butt joint
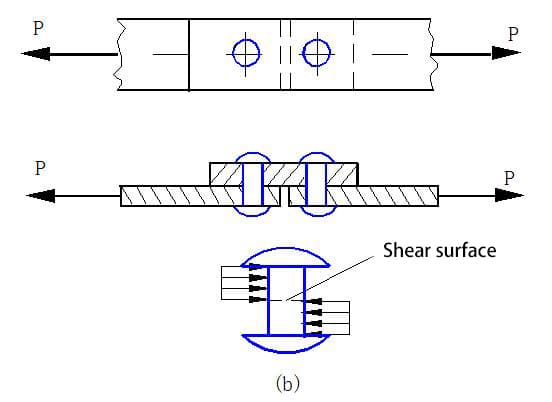
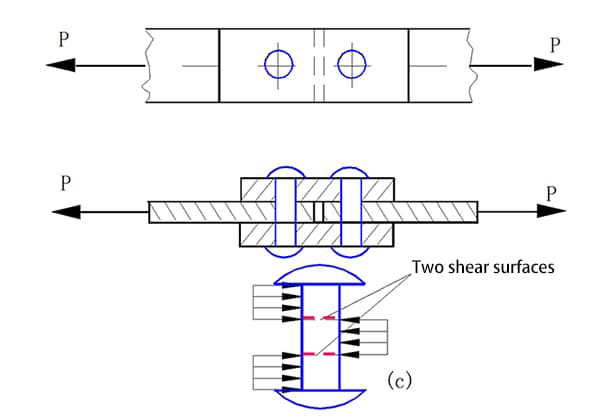
Double cover butt joint
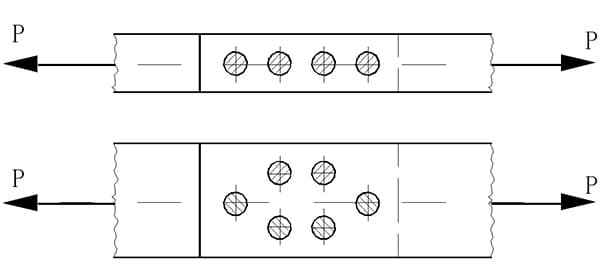
Rivet Group Side Load Bearing
In the riveted joint (as shown in the figure above), to simplify the calculations, assume that:
- Regardless of the riveting method, the bending effect is not considered.
- If the line of action of the external force passes through the centroid of the cross section of the group of rivets and the diameters of each rivet in the same group are equal, then the force acting on each rivet is also equal.
The formula to calculate the force acting on each rivet is:
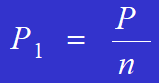
Example:
A joint with four rivets is used to connect two steel plates. The material of steel plates and rivets is the same. The diameter of rivets is d=16mm, the size of steel plate is b=100mm, t=10mm, P=90KN, the allowable stress of rivets is (τ)=120MPa, the allowable yield stress is (σ jy ) =120MPa, and the allowable tensile stress of the steel plate is (σ)=160MPa. Calculate and check the strength of the riveted joint.

(1) Shear strength of rivets:
The force acting on each rivet is P/4.
The shear force acting on each rivet is given by:
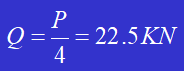
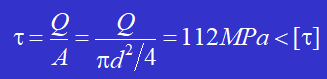
(2) Crushing resistance of rivets:
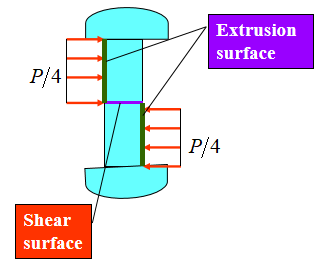
The force acting on each rivet due to crushing is:
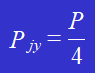
The area of the rivet being crushed is:


(3) Tensile strength of steel sheet

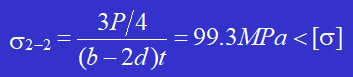
Question to think about:
Shear surface area of dowel pin A.
Ajy guide pin extrusion surface area.
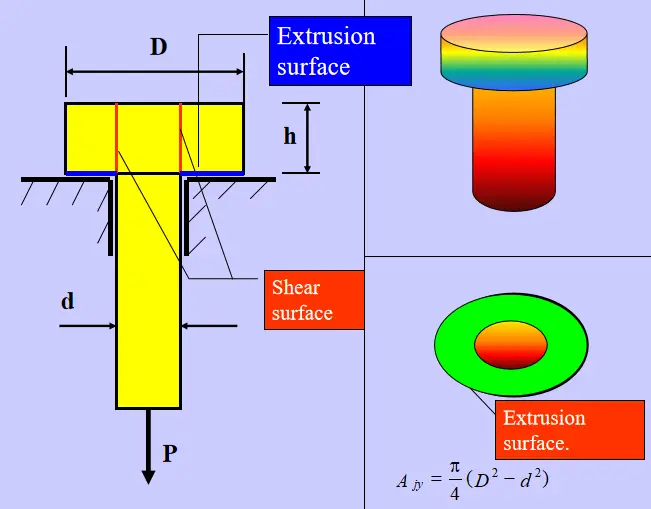
Additional question:
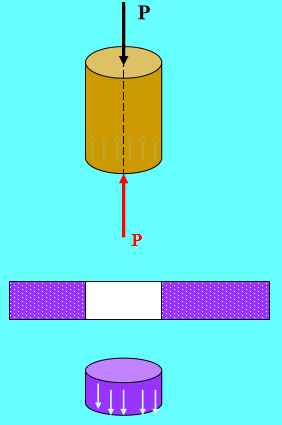
Drill a hole with the shape shown in the figure in a 5 mm thick steel plate. If the shear strength limit of the steel plate material is 𝜏 𝑏 = 300MPa, calculate the punching force F required for the punch press.
Solution: The shear surface area is

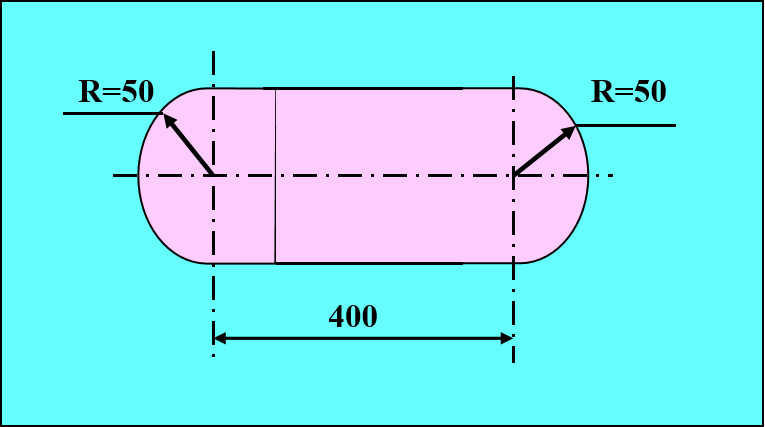
Additional question:
The maximum punching force of a punching machine is P = 400KN, the allowable compressive stress (𝜎) of the punch material is 440MPa, and the shear strength limit of the steel plate is 𝜏𝑏 = 360MPa. Determine the minimum diameter d that the punch can pierce and the maximum thickness 𝜹 of the steel plate that can be punched.
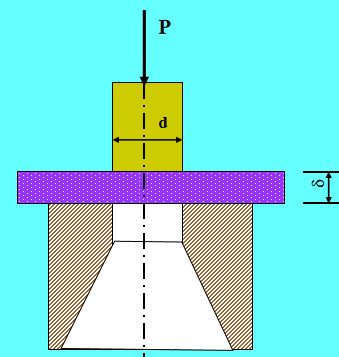
Solution: The punch undergoes axial compressive deformation.
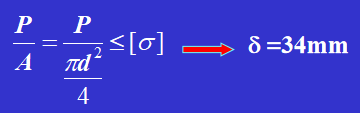
According to the shear failure condition of the steel plate:

Example:
Using two steel rails to rivet into a composite beam, the connection situation is shown in figures a and b.
The cross-sectional area of each steel rail A is 8000 mm, and the moment of inertia of the cross-sectional area of each steel rail about its own centroid is I = 1600 × 10 mm. The rivet spacing s is 150 mm, the diameter is d = 20 mm and the allowable shear stress (τ) is 95 MPa. If the internal shear force Q of the beam is 50kN, check the shear strength of the rivets. Friction between the upper and lower steel rails is not considered.
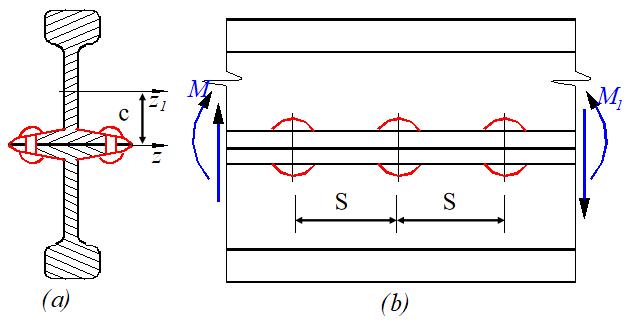
Solution: When the two upper and lower steel rails bend as a whole, the cross-sectional area of the upper steel rail is under compressive stress and the cross-sectional area of the lower steel rail is under tensile stress.

Due to the different bending moments in adjacent cross sections, the normal stress at the corresponding points is different, and therefore there is a tendency for longitudinal displacement along the contact surface between the upper and lower steel rails, causing the rivets to bear forces of shear.
The shear force supported by each row of rivets is equal to the difference in compressive (tensile) force in two cross sections of a steel rail at a longitudinal distance of S.
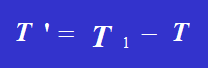
Assuming that the steel rails transmit shear stress across the entire contact surface, the width of the contact surface is b.
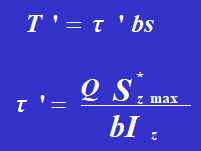
S zmax represents the static moment of the cross-sectional area of a steel rail in relation to the neutral axis.
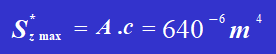
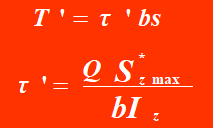
L z is the moment of inertia of the entire cross-sectional area relative to the neutral axis.


The rivet shear stress is:
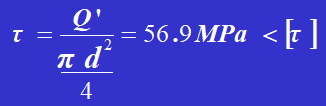
The rivet shear stress meets the strength criteria.
The rivet assembly subjected to torsional loads
Set of rivets subjected to torsional loads (see Figure).
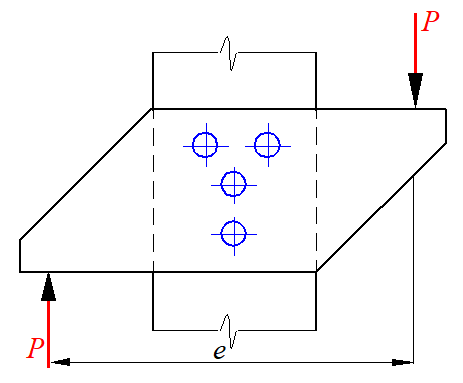
Let the centroid of the cross section of the rivet assembly be point 0.
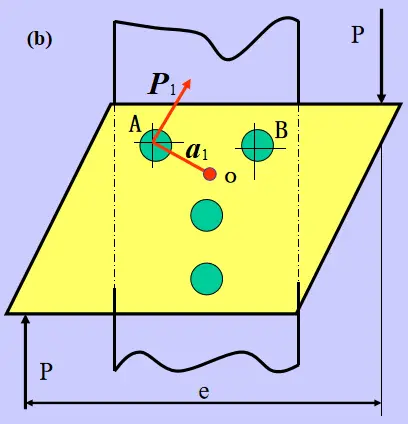
Assuming that any straight line on the steel plate (such as OA or OB) remains straight after rotation, the average shear strain of each rivet is proportional to the distance from the center of the rivet's cross section to point O.
If the diameter of each rivet is the same, the force on each rivet is proportional to the distance from the center of the cross-section of the rivet to the center of the sectional center of the rivet set O, with the direction perpendicular to the line connecting the point and the center O.

P i represents the force acting on each rivet, and a l represents the distance from the center of the cross section of a given rivet to the centroid of the cross section of the rivet assembly, denoted as O.
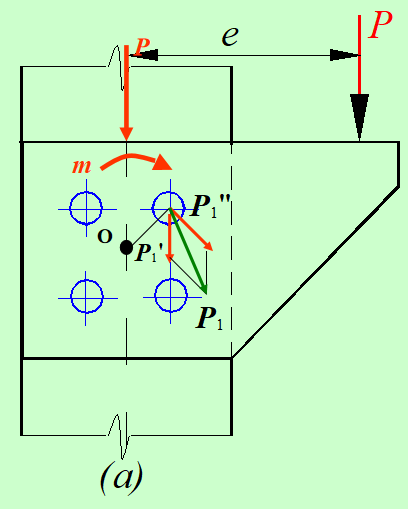
The rivet assembly subjected to eccentric lateral loads (see Figure a).
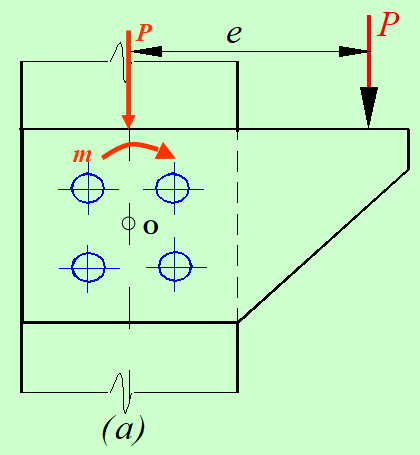
Simplifying the eccentric load P acting on the rivet assembly up to the centroid point O, we obtain a force P passing through point O and a moment m = Pe that rotates around point O.
If the diameter of each rivet in the same set of rivets is the same, the force P1′ caused by the lateral force P and the force P1” caused by the moment m can be calculated. The force acting on each rivet is the vector sum of P1′ and P1”. After determining the force P1 on each rivet, the shear and compression strength of the rivet at maximum force can be checked separately.
Example:
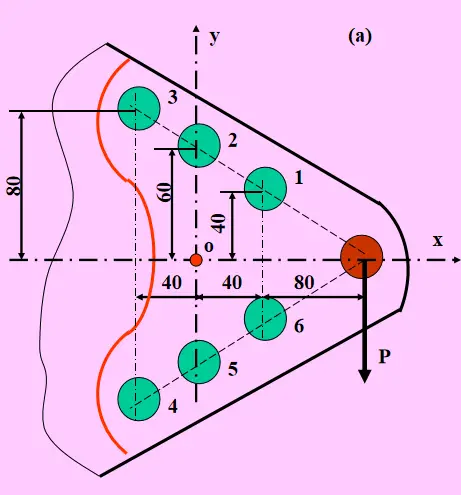
A support connected by a single rivet is subjected to a concentrated force P, as shown in Figure a. The external force P is known as 12 kN. The rivet diameter is 20 mm and each rivet is subjected to a single shear. Calculate the maximum shear stress in the cross section of the rivet under the maximum force.
Solution:
The rivet assembly is symmetrical about the x-axis, and the center of rotation is at point O, which is the intersection point of the line connecting rivet 2 and rivet 5 to the x-axis.
1. Simplifying the force P at point O, we have:
P = 12kN.
m=12 0.12=1.44KN.m
2. Under the action of the force P passing through the center of rotation, and considering that each rivet has the same diameter and material, the force on each rivet is equal.
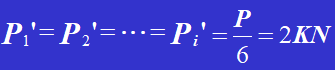
3. Under the action of moment m, the force that each rivet supports is proportional to the distance from the rivet to the center of rotation.

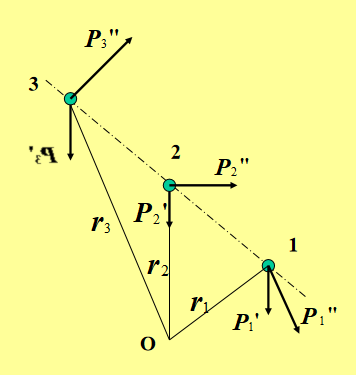
According to the equilibrium equation:
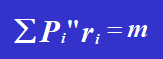

Solving the equation, we obtain:
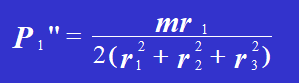

Therefore,
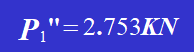


4. Draw the force diagram for each rivet and combine the vectors Pi' and Pi” to obtain the total shear force acting on each rivet, including its magnitude and direction. It can be concluded that rivet 1 and rivet 6 support the maximum force, with the maximum force value being:

The shear stress in the cross section of the rivet is: