1. Overview
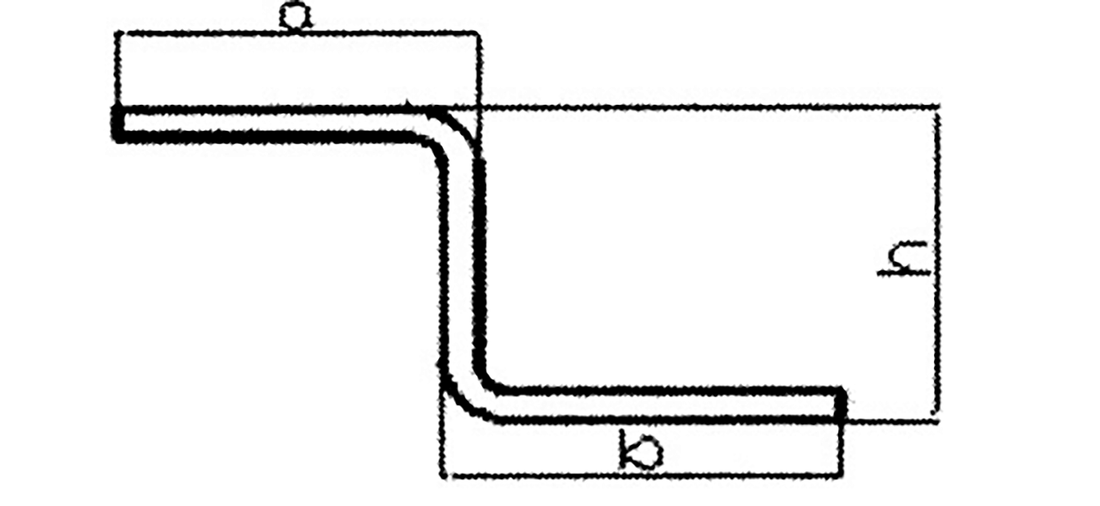
The Z-shaped bent part shown in Figure 1 is a commonly found part in production. The size of h is limited by the lower die, with the minimum size that can be doubled by the existing lower die shown in Table 1.
Table 1 Minimum Size Flexion
| Board thickness | 1 | 1.5 | two | 2.5 | 3 |
| Minimum size | 7 | 9.5 | 14 | 16 | 18 |
In practical production, if there are numerous Z-shaped bending dies smaller than the mentioned size, it would be necessary to design a composite bending die to carry out primary molding. This composite die can be used to bend Z-shaped bending dies of various sizes in different sheet materials.
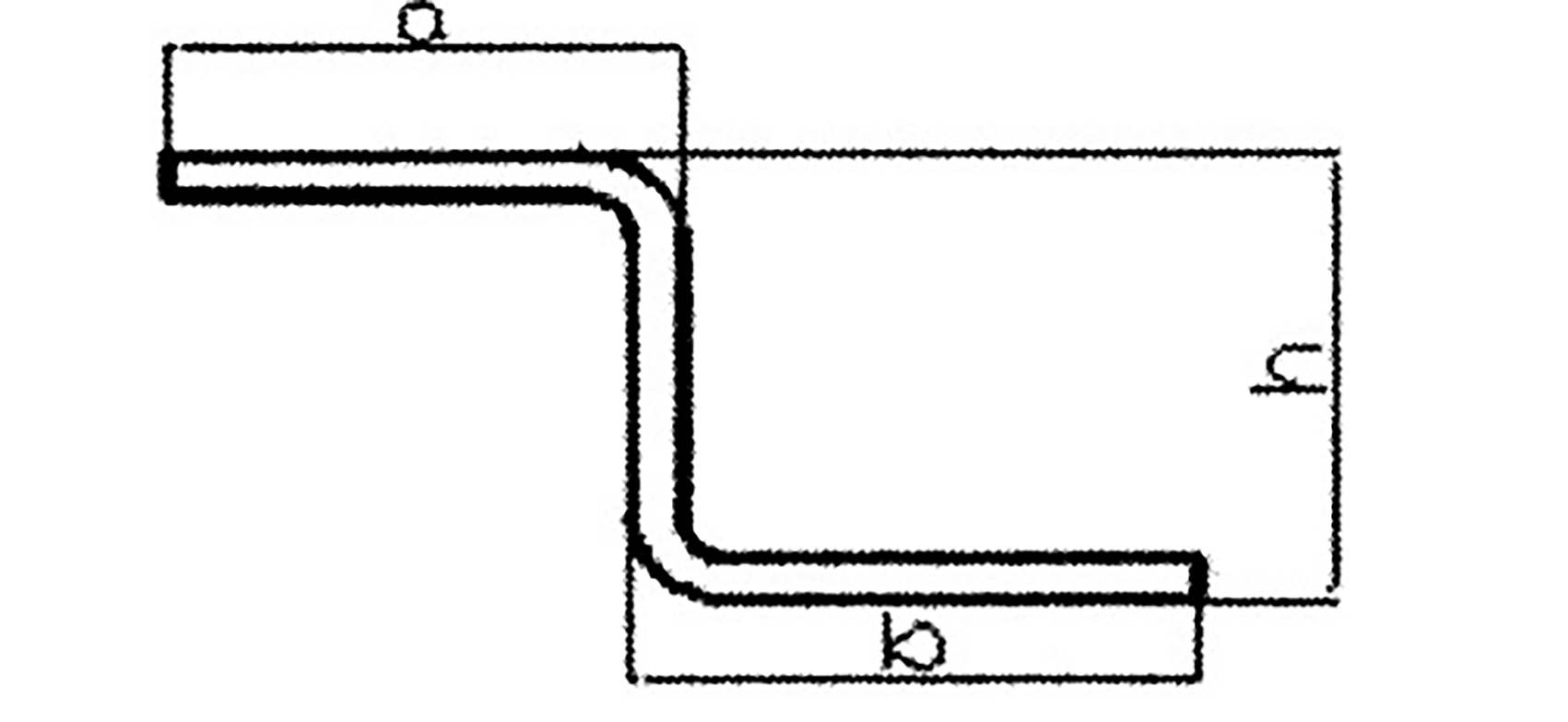
Fig. 1 Z-shaped bending matrix
two . The bending deformation process
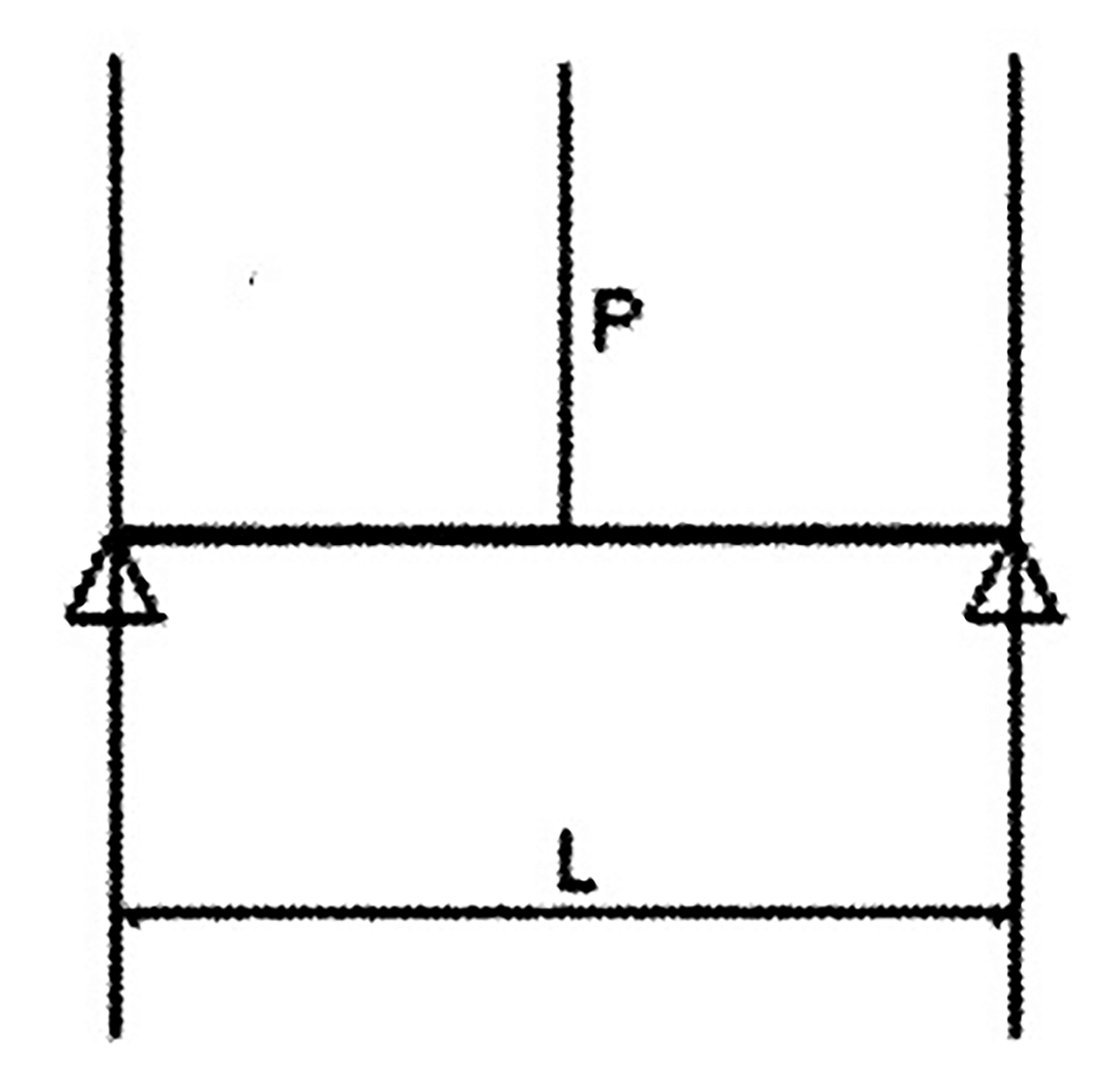
Fig. 2 Action force diagram
As represented in Figure 2, when a metal sheet is subjected to bending, it suffers bending moment, shear force and local pressure. However, the main effect of bending deformation is the bending moment.
The application of external force leads to the corresponding deformation of the metal sheet, which also triggers the appearance of internal force that resists the deformation. The internal force balances with the external force and is measured as tension, which is the internal force per unit area of the object. The greater the external force, the greater the stress and deformation.
When the external stress of the material is below its elastic limit, the sheet metal is in a state of elastic deformation. According to Hooke's law, the linear relationship between stress and strain in the section is due to the linear change in the distance between the strain and the core layer (elongation of the outer layer and shortening of the inner layer).
If the external force is removed, the sheet metal returns to its original shape. However, if the external force continues to increase, the degree of deformation of the bent part will continue to increase until the stress caused by the external force is equal to the yield strength of the material, leading to plastic deformation of the external material.
As the external force increases, plastic deformation progresses from the surface to the center. Once the external force is removed, the elastic deformation immediately disappears, but the plastic deformation remains and results in permanent bending deformation.
If the stress caused by the external force exceeds the resistance limit of the material, the metal sheet suffers a fracture due to plastic deformation. Internal compression during bending of sheet metal also produces plastic deformation, but this type of plastic deformation increases surface stress without causing damage and is therefore often ignored.
3 . C bending calculation method
Now we carefully observe the plastic bending deformation.
Under the action of the bending moment, there are three equal lines in the plate section: ab= a 1 b 1 = a 2 b 2 .
After bending, the inner layer shortens and the outer layer lengthens, i.e. ab < a 1 b 1
Therefore, during bending, the inner material is subjected to compression and becomes shorter, while the outer material is stretched and lengthened.
Between tension and compression, there is a layer of material that undergoes neither stretching nor compression and is called the neutral layer. This layer remains unchanged in length and does not lengthen or shorten.
The process of calculating the bent part involves dividing it into several basic geometric elements, including straight line segments and arc segments. The length of each element is calculated individually and the total length of all elements is the unfolded length of the folded part.
The Z-shaped bent part in Figure 1 can be divided into five units as shown in Figure 2. Units 1, 3, and 5 are straight line segments, while units 2 and 4 are arc segments.
As discussed earlier, the fiber layer with constant length in the middle before and after bending is called the neutral layer. When you calculate the expansion length of the arc segment, you are actually calculating the neutral layer length of the arc segment.
The position, x, of the neutral layer on the inner side of the arc (as shown in Figure 3) is generally determined by the ratio r/t.
x =kt
Where:
- t — Material thickness
- k — Neutral layer position coefficient (or neutral layer coefficient)
- k = R – r/t
- R = r +kt
Where:
- R — The distance from the center of radius r to the neutral line of bending
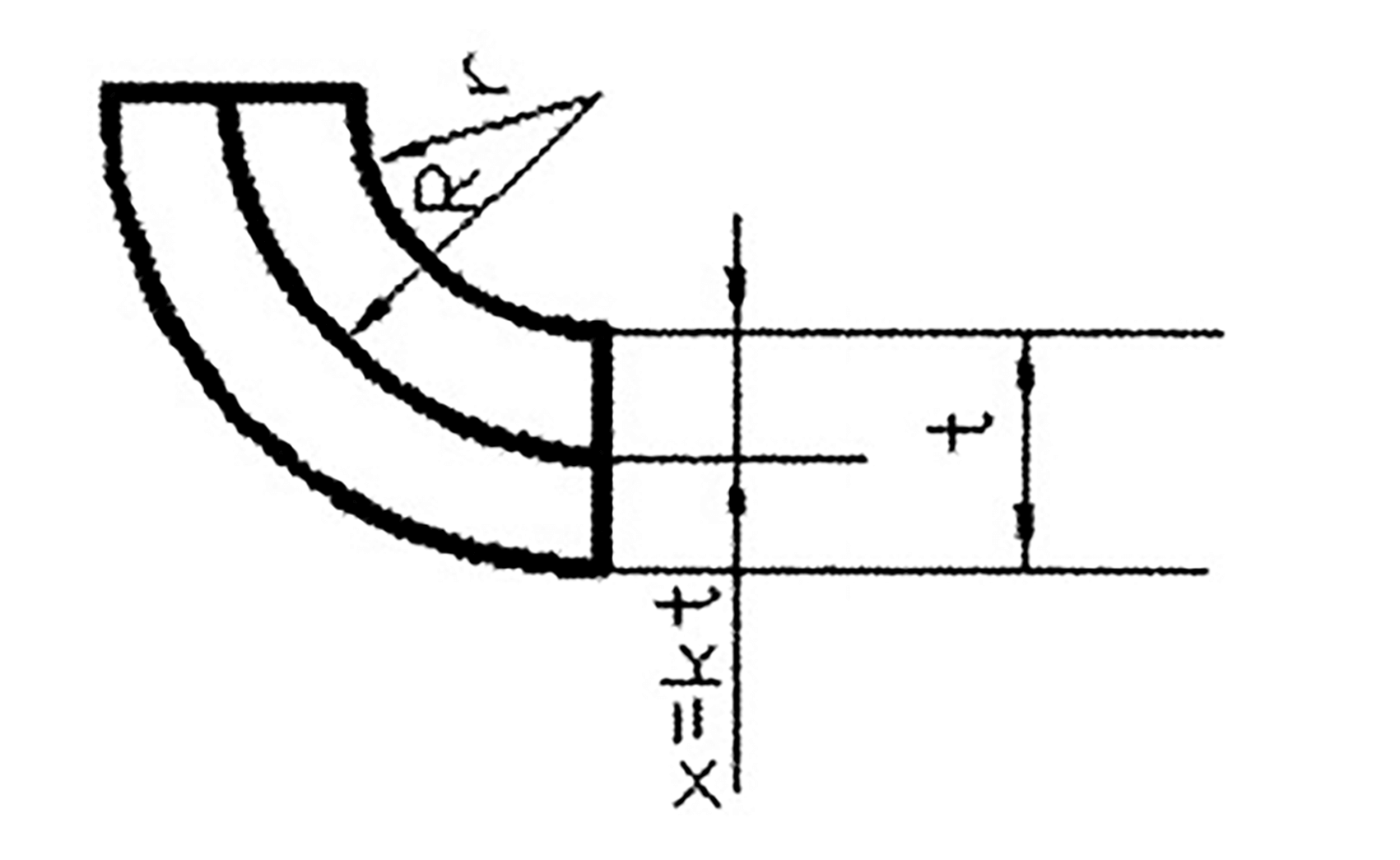
Figure 3
The value of k varies with the relationship between the internal radius and the thickness of the sheet, shown in Table 2:
table 2
| r/t | 0.25 | 0.5 | 1 | two | 3 | 4 |
| k | 0.26 | 0.33 | 0.35 | 0.375 | 0.4 | 0.415 |
In practical production, it is more common to bend steel plates into a 90-degree shape.
Calculation of arc length for 90 degree bending with different ret values can be obtained through reference tables in practical applications.
This article focuses on 90-degree right-angle bending, but it is not efficient to calculate the straight line and arc segments separately for 90-degree sheet metal bending parts. Instead, they are marked as shown in Figure 1.
When calculating the unfolded material, we can directly use the marked dimensions to simplify the calculation process.
According to figure 1, the length of the material developed is calculated:
L = a+b+h – 2x
Where:
- x — Common bending coefficient
4. Structure and working process of Z-shaped bending composite die
Table 3 Common bending coefficient x
| t plate thickness | Internal radius of curvature r | |||
|---|---|---|---|---|
| 1.0 | 1.5 | 2.0 | 2.5 | |
| 0.5 | 1.0 | 1.2 | 1.4 | 1.6 |
| 1 | 1.9 | 2.1 | 2.3 | 2.5 |
| 1.5 | 2.5 | 2.7 | 2.9 | 3.1 |
| 2.0 | 3.4 | 3.6 | 3.8 | 4.0 |
| 2.5 | 4.0 | 4.2 | 4.4 | 4.6 |
| 3 | 4.9 | 5.1 | 5.3 | 5.4 |
This simple set matrix is different from the conventional matrix.
It is designed to be simple, fast, easy to process and straightforward to form. Although it may not be as accurate as conventional die, it is still used to process products quickly and accurately.
The composite matrix formation diagram is shown in Table 3.
Processing principle: The thickness of the joint is adjusted to achieve the desired width of the V-groove in the upper and lower die and to realize one-time Z-bending processing under pressure.
Die structure: The Z-shaped bending die consists of an upper die, a lower die, a gasket and an angled prismatic steel.
Joint Thickness: The spacer is made of 0.5mm thick steel and is stacked to achieve the required thickness.
Prism Steel: A rectangular steel piece within the die, its four angles are chamfered into sides of 0.5mm, 1.0mm, 2.0mm and 4.0mm, as shown in Table 3.
The special simple die is used to achieve the desired V-groove width of the upper and lower die by adjusting the angle prism steel size and gasket thickness, and then carrying out Z-bending processing in a press.
This method is chosen because of the potential to increase the V-groove and reduce creasing, so different plate thicknesses require different teeth, as shown in Table 4.
Table 4
| Board thickness | t<0.8 | 0.8 |
1.0 |
1.2 |
t>1.5 |
| Tip | 0.5 | 1.0 | 1.0 or 2.0 | 2.0 | 4.0 |
Z-shaped bending die debugging method:
1) Both folds of the straight edge Z fold are 90°. The distance between the two ends of the tool is: 1.414/2×h;
2) If the crease of the piece is very deep, you will need to choose a large angle.
b: iron; c: increases the angle R;
3) If the height is reached, but the angle is greater than 90°, then the: eccentricity of the matrix.
b: Increase the thickness of the shim;
4) If the two sides of the Z bend are not parallel, this can be achieved by increasing or decreasing the thickness of the shim.
If the upper bend is greater than 90°, the thickness of the lower die shim needs to be increased; and if the lower bend is greater than 90°, the thickness of the upper die shim needs to be increased.
Z curvature expansion calculation method:
When h > normal fold size, it should unfold into two folds.
l = a + b + h – 2x
Where:
- l – Length of unfolded material
When h < the normal bending size, it is expanded by one-step forming.
L = a + B + h – 1.5x
Where:
- x — Common bending coefficient
One of the empirical formulas in practice is to subtract 1.5x from the total dimension of a frame.