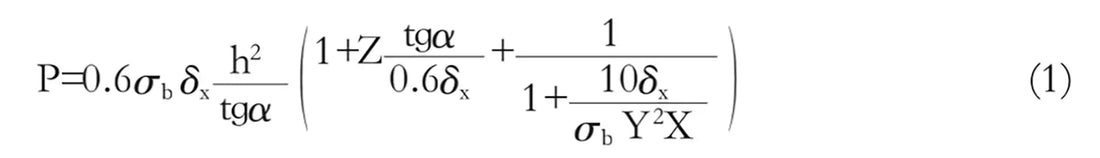Swing plate shears are widely used because of their simple structure, low failure rate, high cutting efficiency and ability to prevent plate sagging, warping and distortion after cutting, unlike ordinary plate shears. This is mainly because they mainly adopt an oblique cutting edge.
During the cutting process, the rotating shear turret rotates, causing the blade angle and clearance to change. However, in the design process, the calculation of the shear force of the swing beam shear is often based on the straight-line motion shear force calculation formula of the blade support. This can lead to inaccurate calculations, deviations from the project size and further affect its normal performance.
1. Calculation of shear force
The calculation of shear force in oblique blade cutting machines that move the tool holder in a straight line mainly uses the Nosari formula, which was proposed by scholars from the former Soviet Union.

In the formula:
- σ – Resistance limit of the sheet to be cut, N/mnm;
- δ – Ductility of the sheet to be cut;
- H – Thickness of the sheet to be cut, mm;
- α – Shear angle, degree;
- X, Y, Z – The bending force coefficient, relative value of the shear blade lateral clearance and material pressure coefficient.
Clearly, formula (1) does not take into account the shear process after changes in the shear angle, and the shear clearance is also considered based on a single value. As a result, it is only suitable for the knife structure that performs linear motion shearing.
2. Shear angle and shear clearance
In swing-type plate shears, to maintain a constant shear clearance and shear angle during the shearing process, the blade mounting surface on the tool holder must be machined into a spiral surface in space.
However, in practice, to simplify the machining process and take into account the shape of the blade (which is usually rectangular with a flat back surface), the spiral surface is machined directly in a plane parallel to the axis of rotation of the blade. toolholder.
As shown in Figure 1, there is an ideal installation position at AA' and an actual installation position at BB' (where Ф represents the shear angle in the figure).
2.1 Shear angle
Because the tool is not installed according to the spiral surface, the current installation method involves making the blade tangential to the installation surface at a certain height.
This means that when the blade is mounted on the tool holder at a certain shear angle Ф, the blade is always tangent to AA' (as shown in Figure 1) due to the tightening of the screws.

Fig. 1 Installation diagram of the swing plate scissor blade
To ensure shear quality, the swash plate shear is elevated above the y-plane of the table's rotating axis to maintain a constant clearance angle γ during the shearing process.
However, because the blade mounting plane is parallel to the rotation axis of the tool holder, a certain blade thickness and height can only be installed at BB' or parallel to it.
As shown on the left side of Figure 1, the blade changes from the ideal position tangent to point A to the BN position tangent to point N. This results in a change in the shear angle as shown in Figure 2.
When the shear point is moved from point M to point B, the shear angle gradually changes from γ (which needs to be kept at a constant value) to γ = γ' + β.
When the shear point moves from point B to point M, γ' = γ – β, as shown in Figure 2 (a).
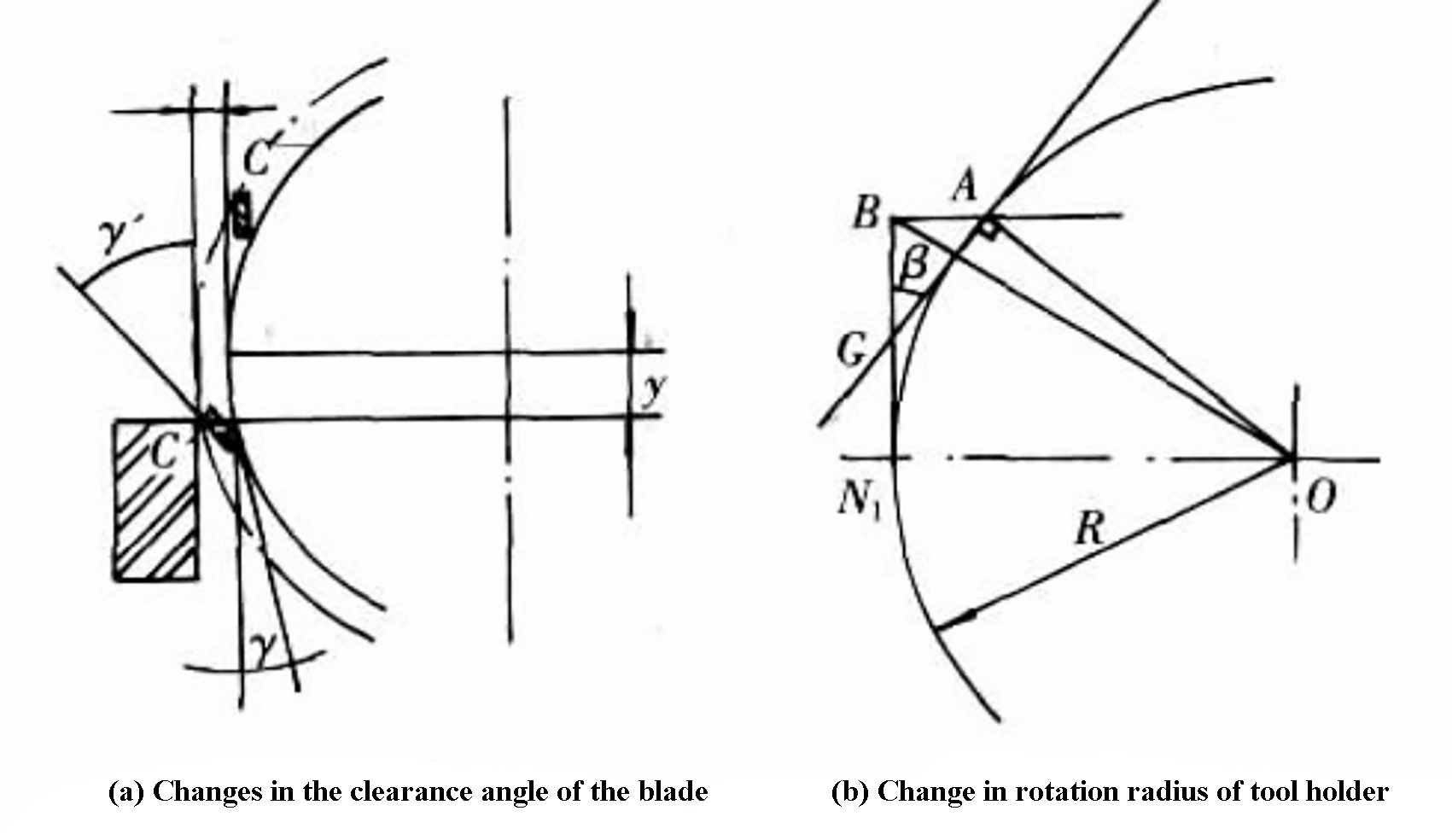
Fig. 2 Change in angle and radius of rotation of knife edge in swing plate shear processing
If the length of the shear blade is l, the shear angle is Ф, and the radius of rotation of the blade is R, the geometric relationship shown in Figure 2b is obtained when the entire blade is used.

If n blades are used and the thickness is adjusted using adjusting shims, like this:

From the total length, it can be seen that the range of the back angle of the blade during cutting is γ ± β.
2.2 Shear clearance
To facilitate installation and improve shear quality, swing plate shears generally use long blades.
When the blade is installed in the tool holder along the axis direction with a shear angle Ф, if the shear point moves from B' to B (Figure 1) during the shearing process, the actual radius of rotation of the tool holder will increase.
Assuming the radius of rotation is R, the shear angle is Ф, and the length of the tool holder is l, the difference between the maximum radius of rotation OB and R is shown in Figure 2(b):

To ensure that the cutting edge does not damage the table during the shearing process, it is necessary to maintain a shear clearance △ greater than △R.
Taking QC12Y-6×200 swing type plate cutting scissors as an example, with rotation radius of R = 469 mm, shear angle of Ф = 1.5° and blade length of 1100 mm with 3 pieces, replacing these values in formulas ( 3) and (4) give β ≈ 5° and △R = 1.87mm, respectively.
3. Impact
During the plate shearing process, the shear quality and strength are highly dependent on the shear gap. The effects of shear and pull-off coexist during the process, and the increase in shear gap results in a higher proportion of pull-off, but at the same time, the shear quality worsens.
For shearing thick plates, the shear clearance should generally be controlled within the range of 8% to 12% based on experience. However, the use of simplified swing-type scissor blade mounting surface technology makes it difficult to achieve this requirement.
When the shear gap exceeds the empirical value, it will inevitably lead to changes in shear force. Equation (1) shows that an increase in the shear gap leads to an increase in the relative value of the lateral gap of the shear blade, which ultimately results in an increase in the shear force required during the shearing process.
During the shearing process, the shearing action will increase the shearing movement in two ways:
Firstly, it will increase the shear force, resulting in greater power loss.
Secondly, it will increase the plastic deformation of the plate, leading to increased friction between the blade and the shear plate. This will increase the shear force required for shearing and reduce tool life.
Therefore, for swing-type plate shear, it is appropriate to choose a relatively larger value of the shear blade side clearance and blade wear coefficient when using formula (1) to calculate the shear force, in order to compensate the impact of the above factors.
To ensure the shear quality and avoid plate friction between the blade and the back surface of the blade, the design of the swing-type plate shear requires that the angle between the back blade and the vertical surface of the table is 1.5° at 2.0° during the shearing process.
Based on the above analysis, the change of shear incidence angle of rocker-type plate is γ ± β during the shearing process.
After calculating β ≈ 5° in the shear of the QC12Y-6×200 sway beam, it is difficult to guarantee the design requirements of the back angle. In the shearing process, even a negative back angle can intensify blade wear and heat, and even produce extrusion, reducing blade strength.
To avoid this situation, a fixed angle θ between the front of the blade and the vertical surface of the table is required in the design of the swing-type plate shear tool holder (see Figure 3). As long as θ is greater than or equal to -β, there will be no compression phenomenon between the blade and the plate. This angle is not described in some documents and is recommended to be 5° to 7°.
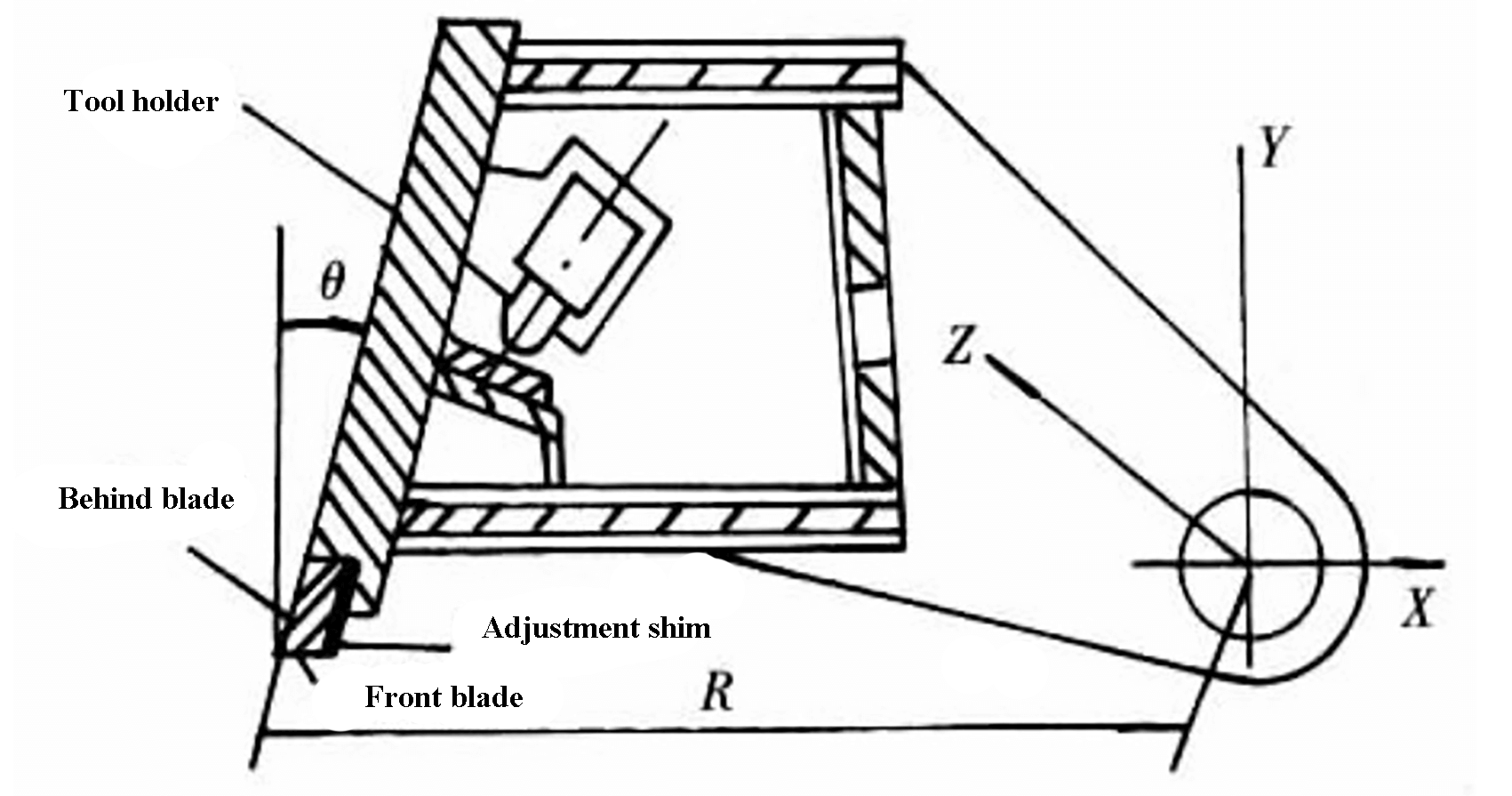
Fig. 3 Schematic diagram of the hydraulic rotary scissor blade structure
4 . Conclusion
Calculation of shear force in swing-type plate shears is typically done using the tool holder to obtain linear motion in the formula for oblique blade shear.
Although theoretically possible, the actual machining process involves simplifying the blade mounting surface from a spatial spiral surface to a flat one. This results in changes in the shear clearance and shear back angle during the shearing process.
Changes in shear clearance can affect the shear force and decrease the quality of the shearing process. Conversely, changes in the back angle after shearing can cause wear and even extrusion between the blade and plate, ultimately increasing the shear force.
At present, the simplified process is commonly used in manufacturing the blade mounting surface of turntable type scissors. Therefore, it is essential to consider the influence of shear clearance and change in back angle when calculating shear force.