This article deals with the design of steel columns in accordance with Eurocode 3, EN 1993-1-1. Each project and design process is explained in detail.
Steel columns are designed to withstand axial compression and bending stresses caused by fasteners. Unlike concrete, steel is weak in compression. There are basically two types of failures in steel columns.
- Material crushing – material failure
- Buckling of components (general buckling or local buckling)
Material crushing
The material reaches its capacity limit and fails because it can no longer withstand the applied load.
This type of failure may be due to insufficient compressive strength and/or cross-sectional area.
Buckling of bars
General or localized bending can lead to design defects in steel columns. Local bending depends on the cross-sectional properties.


Overall buckling depends on several factors.
- Column length
- Restraints at each end (untied, partially tied, and fully tied)
- Cross-section properties (cross-sectional area, shape, etc.)
For more information, see the article on Column Failure Methods for Different Types of Defects in Concrete Columns.
Let us discuss the design aspect of axial load column.
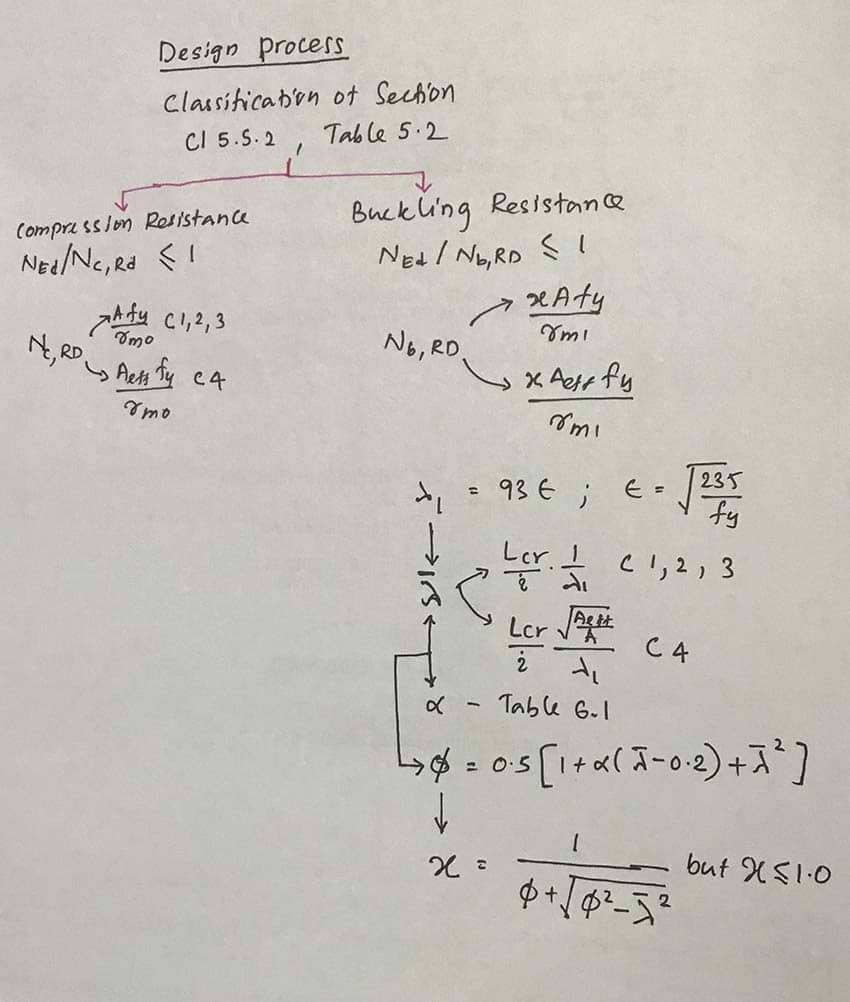
Steel Column Design Method
- Section classification
First, the cross section must be classified according to the cross section dimensions and material properties.
Based on the slenderness limits, the profiles are divided into the categories Class 1, Class 2, Class 3 and Class 4. Therefore, the profiles in Classes 1, 2 and 3 are classified as non-thin profiles and the profiles in Class 4 as thin profiles. .
More information can be found in section 5.5.2 of EN 1993-1-1 (EC3).


Let's take a look at the H section and see what limits are available to classify the section. The limits are as follows, as shown in Table 5.2.
| Relationship | Class 01 | Class 02 | Class 03 | |
| Laminated profile pressure flange | C F / T F | 9ε | 10ε | 14ε |
| Bridge made of laminated profile | C I /T I | 33ε | 38ε | 42ε |
Where ε = √(235/f j )
-
Plastic resistance or transverse resistance, N c, Road
- Non-thin profiles: Class 1, 2 and 3
N c,Rd = Af j /γ m0
-
- Thin section: Class 4
N c,Rd = ON ef F j /γ m0
One and One and f must comply with Sections 6.2.2.1 and 6.2.2.5.
After calculating the resistance, the following capacity test must be carried out.
N Ed. /N c,Rd ≤ 1.0
- Buckling resistance, N b, Road
First calculate the buckling resistance depending on the section class.
-
- Non-slender section (Class 1, 2 or 3)
N b, Road = χ A f j /γ m1
-
- Narrow cross section (Class 4)
N b, Road =χA ef F j /γ m1
If A and A and f The holes determined for fixings at the ends of the supports do not need to be taken into consideration.
Where “χ” is the buckling reduction of the section. It can be calculated using the following equation.


α is an imperfection factor that can be found in Table 6.1 of the code below.
| Bend Curve | To 0 | A | B | W | D |
| Imperfection factor α | 0.13 | 0.21 | 0.34 | 0.49 | 0.76 |
Here a 0 a, b, c and d are buckling lines to select from Table 6.2: Selection of buckling line for cross sections according to EN 1993-1-1. The part of Table 6.2 that gives the curves for rolled sections is shown in the following figure.


A simplified method for calculating λ‾ is presented in Section 6.3.1.3 of EN 1993-1-1.


- Check N Ed. /N b, Road <1
The design process flowchart can be shown below.

For further explanations about the calculation process, you can consult the other article published on EC3 with a worked example.

