The forces acting on a dam must be considered from the planning phase until its completion. This is important not only for the construction, but also for the usability of the dam.
During the construction of the dam, instrumentation systems were installed to monitor its condition.
It is recommended to read the article Types of dams. More information about the different types of dams, their characteristics, dam instrumentation and the different measurements used to monitor dam stability can be found here.
This article discusses the following types of forces acting on a dam.
- Water pressure
- buoyancy pressure
- Wave pressure
- Earthquake forces
- Wind pressure
- Mud pressure
- Thermal loads
- Ice pressure
- own weight
Let's discuss each type of force applied to a dam in detail. This discussion describes the design and construction aspects of applying load to a dam. Furthermore, we discuss the type of load exerted due to the function of the dam. For example, mud pressure can be classified in this range.
Let's look at each type of load individually.
Water pressure
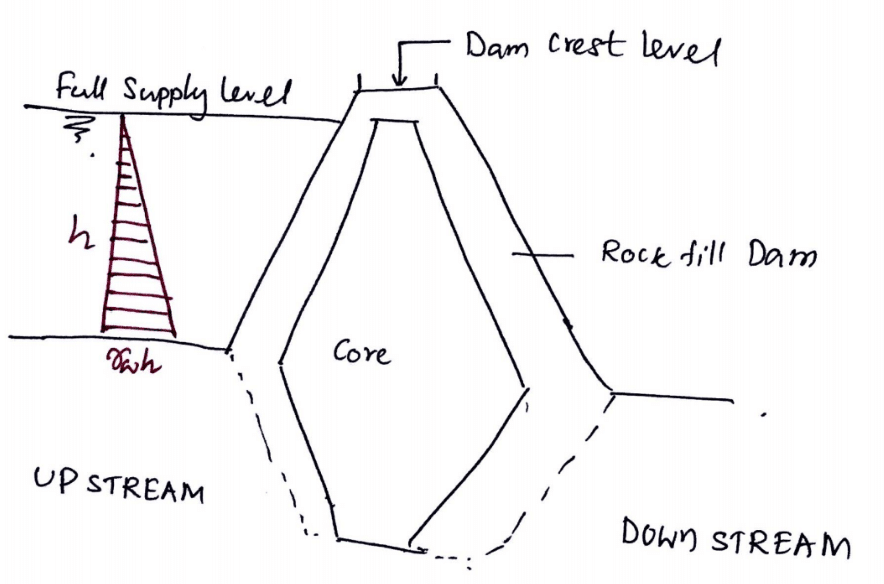
Dams are built primarily to retain water. Therefore, they must be constructed in such a way that they can withstand water pressure.
The well-known water pressure equation is as follows.
P = γ I H
Where P is the pressure (kN/m 2 ), γ I is the density of the water (kN/m 3 ), and H – is the height of the water.


The figure above shows the effect of water pressure on a dam filled with rocks. Similarly, water pressure could be calculated for other types of dams.
From the numbers above we can calculate the force (F) exerted on the dam as follows:
F = 0.5γ l H 2
And the forces act at a third of the height we consider the height of the water.
In addition to the points above, the maximum water level must be taken into account in the project. Generally, two types of water levels are considered in dam design.
- Total Supply Level (FSL)
- Maximum Floor Height (MFL)
Total Supply Level (FSL)
This is the operating level of the dam. We generally maintain water levels at maximum supply levels.
The height of the dam will be above this level. Additionally, all gates must operate at this level to meet dam design requirements.
For dams built exclusively for power generation, the water level is maintained at this level and if a rise is observed, more power is generated to maintain the water level in the FSL rather than passing the water over the spillway.
Maximum water level (MWL)
The maximum water level is the water level that the dam reaches during floods due to the water level rising above the FSL.
The dam must be able to withstand the rise in water level to the maximum water level and the spillway gates must be able to release the water without the water level rising above the maximum water level.
It is planned to operate the gate between FSL and MWL and maintain the maximum water level as MWL during operation.
Furthermore, and most importantly, the dam must also be designed for this water level. When considering the forces acting on a dam, it is important to ensure that the elevation of the water to the maximum water level is taken into account to check stability.
buoyancy pressure
The movement of water below the body of the dam due to seepage creates buoyancy pressure in the dam.
The stability of the dam is checked for tipping, sliding and loading.
The buoyancy pressure on the embankment affects the tipping safety factor and the load capacity. The impact on bearing capacity may vary depending on the type of ground on which the foundation is built.
Dams can collapse due to the lateral and buoyant forces exerted by the water. Therefore, the buoyancy forces acting on a dam are considered very critical loads.


As shown in the figure above, the water level under the foundation varies. Depending on upstream and downstream water levels.
The water pressure in the inspection tunnel fluctuates due to the reduction in water pressure. This may be due to the joint curtain or because water can drain through the drainage tunnel.
The figure above shows that water can flow through the drainage tunnel to reduce the buoyancy pressure on the dam.
Additionally, injection curtains can also be constructed to prevent water movement under the dam body. Furthermore, curtain injection is increasingly used in dam construction to minimize water infiltration.
Variation in tailgate height must be carefully considered in the design as it significantly affects lifting pressure. The case of heavy loads must be taken into account in the design.
If there is no drainage tunnel or grouting curtain, the design must take into account the variation in fall between the upstream head and the downstream head.
Wave pressure
Waves on the water surface caused by wind put additional pressure on the dam.
There are different methods to calculate the pressure in the dam. One of the methods we could use is explained in this article.


H i = 0.032√VF + 0.763 + 0.271(F)3/4 for F < 32 km
H i = 0.032√VF for F > 32 km
where; H I – height of the wave in meters, V – wind speed in km/h, F – extension of the wave in a straight line in km
The maximum pressure Pw can be calculated as follows.
Pw = 2.4γ i H i works via h i /2 above the still water level
Furthermore, the pressure distribution is considered triangular and has a height of 5h l /3
Therefore the total force is (F i ) due to wave movements can be calculated as follows:
F i = 0.5 (2.4γ i H i ).5h i /3 = 19.62 hours i 2
The force acts 3hw/8 above the water level in the reservoir as indicated in the figure above.
Earthquake forces
Depending on the location and return period, the magnitude of an earthquake is selected when designing dams.
A dam is such an important structure that it costs a lot of money. Therefore, it must be safe enough to withstand the loads placed on it. There should be no uncertainties in the design. Therefore , regardless of whether or not the dam is located in an area prone to earthquakes, the seismic forces acting on the dam are taken into account in the design.
Depending on the categorization of earthquake-prone areas, there are zones with certain seismic intensities. They are based on a probability or the payback period.
The specified resistance must be used if it corresponds to the return period considered in the project. When a high or low return period is considered, the appropriate seismic magnitude must be considered in the dam design.
There are two methods for calculating the seismic resistance of dams and structures.
- Manual method or simplified method with initial force
- More detailed analysis manually or with computer-based software
Manual method or simplified method
As we know the seismic coefficient based on the data to be considered, we can calculate the acceleration.
If we know the acceleration and mass of the structure, we can easily calculate the force.
Force = mass x acceleration
If we know the lateral force created by the earthquake, we can calculate the tipping moment and factor of safety.
This is a method of approximating the structure with a concentrated mass. Therefore, a more accurate method needs to be used to obtain correct answers.
Detailed analysis of earthquake forces
This can be done manually or using computer-based software.
Once the magnitude of the earthquake or seismic acceleration has been selected, we can calculate the forces acting on the structure according to the guidelines provided in the code.
Different types of methods for calculating earthquake forces are discussed in the article lateral loads according to UBC 1997.
Or computer software could be used to calculate the stability of the dam. Provides the dam safety factor. These methods used for rockfill dams, as well as the manual method, are very difficult to calculate the slope stability safety factor.
The software allows you to determine the most critical safety factor for different types of failures.
| project status | Minimum safety factor |
| Operating base earthquake | 1.3 |
| The largest credible earthquake | 1.1 |
Furthermore, the lady's safety factor is checked on several occasions as mentioned in the table above.
Water pressure caused by earthquakes
Water pressure fluctuates during an earthquake. He is not static.
The following figure shows the effect of water pressure during an earthquake.
The figure above shows the water fluctuation caused by the earthquake. This pressure must be added to the static pressure to determine the final load.
The article Water pressure caused by earthquakes For more information about the forces acting on a dam based on pressure load calculations, see.
Wind pressure
Wind pressure is generally not taken into account when building dams.
When the dam is full, a lateral load acts on the dam that is much greater than the wind load.
However, a portion of the dam is exposed to wind beyond full supply levels. If necessary, we can take this height into account when applying the wind load.
Mud pressure
Sludge deposits inevitably occur in reservoirs. They reduce dam depth and water storage.
Furthermore, this also overloads the dam.
During floods, mud enters the reservoir.


The underwater pressure exerted by the mud can be calculated as shown in the figure above.
P=k A γ'h
where k A – active earth pressure coefficient, γ' – density of the submerged silt and h – height of the silt deposit.
The force can be calculated as follows.
F = 0.5k A γ'h 2
As mud pressure represents additional pressure placed on the dam, it must be taken into account in the design. Mud can significantly affect the safety of dam tipping if the dam is required to have a higher mud fill height.
Therefore, a special assessment must be carried out to determine the forces acting on a dam by suspended solids.
Thermal loads
Thermal loads are a crucial criterion for concrete dams.
During and after construction, the effects of temperature fluctuations must be monitored. If the limits are exceeded, there are problems Concrete durability .
The rise of concrete due to heat of hydration must be limited in concrete structures to avoid cracking. The article, Methods for limiting the temperature of concrete For more information you can contact us.
In addition, attention should be paid to thermal cracks due to fluctuations in the temperature of the concrete. The article, Thermal Cracking in Concrete provides comprehensive information on this topic.
When calculating the concrete crack width, the following load combinations must be taken into consideration.
- M+T+T2
- T1 only
- T1 + T2
Where,
M – bending moment
T – traction force
T1 – Increase in temperature in relation to ambient temperature due to heat of hydration
T2 – Seasonal temperature deviation in relation to ambient temperature
Increasing tensions
When a dam is dammed, it is filled for the first time.
For rockfill dams, earthen dams and similar dams, impoundment is extremely critical.
As the water level rises, saturation causes stress on the core.
Therefore, there are restrictions on the fill rate. This may depend on the project specification.
A gradual increase in the water level in the reservoir is allowed.
Ice pressure
In countries with seasonal temperature fluctuations, water turns to ice.
As they melt and expand, pressure is applied to the dam.
Could be in the range of 250 – 1500 kN/m2
own weight
The self-weight of the structure must be taken into account when designing stability in the limit state of use and in the ultimate limit state.
The self-weight of a dam can be calculated very easily: It is calculated from the density of the concrete multiplied by the volume of the concrete.
However, if we need to calculate the weight of the rockfill dam, more effort will be required because there are different types of layers with different densities.