Lateral torsional buckling is the deformation of the beam in relation to its longitudinal axis due to applied loads. Furthermore, it causes failure of steel beams.
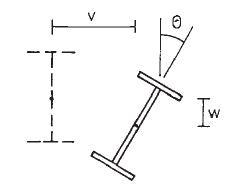
Deformation can occur as translational and rotational movement of the section. These types of movements are called flexural-torsional buckling. Figure 1 shows the deformations that can occur as a result of flexural-torsional buckling.


Figure 01 Bending and twisting
As shown in Figure 01, the beam can be decoded by applying loads. This deformation can occur when the component is rotated laterally and vertically. When designing steel beams, lateral supports are provided at calculated intervals to prevent failure.
Lateral torsional buckling occurs with increasing loads based on cross-sectional properties and their limitations. Loads on a beam cannot be avoided because that is the purpose of the beam.
However, cross-sectional properties and constraint conditions can be controlled during the construction and design phase.
- As explained above, flexural and torsional buckling occurs when the beam is not fully restrained in the lateral direction along the beam's compression flange.
- The beam is considered fully laterally fixed if the connection between the beam and the floor can withstand at least a lateral force of 2.5% of the maximum force in the compression flange of the beam.
If there are no restrictions, sections with a higher section module must be provided. If constraints are provided properly, the size of the conveyor can be reduced.
It has been proven that during failures the moment of the section moves away from its axis. Therefore, by providing limits, the section dimensions will definitely be reduced.
However, due to the structural layout of the structure, it may not be possible to provide lateral limits at the ends or inside. In these situations, the beams must be designed without considering lateral limitations.
Mainly, the lack of fixation of the pressure flange leads to lateral movement of the section. Therefore, torsional buckling can be prevented by internal braking.
Intermediate supports are provided to reduce the unsupported length in the lateral direction. They must withstand lateral forces and have the ability to maintain them without deformation. The axial load capacity of intermediate supports should be checked in accordance with BS 5950 guidance.
Design of flexural-torsional buckling of a beam
To meet bending requirements, the section must have a bending capacity in the bending direction (Mc) greater than the applied bending moment and a lateral torsional buckling capacity greater than the moment generated by the buckling.
M X < M b /M LT and M X ≤M C
This article explains the procedure to be followed in calculating lateral torsional buckling capacity. And the article Construction of steel beams to BS 5950 Bending capacity tests may be required.
Torsional buckling resistance in bending (M b /M LT ) can be calculated as shown below. Two methods for calculating the buckling moment of resistance (M b ). Either method can be used depending on the designer's preference.
- Rigorous method
- Simplified method
| Rigorous method | Simplified method | |
| Class 1 – Plastic Class 2 – Compact |
Mb = PbSX | Mb = PbSX |
| Class 3 – Semi-compact | M b =P b Z X or M b =P b S x,efe |
Mb = PbZX |
| Class 4 – Slim | M b =P b Z x,efe | |
| P b based on λ LT and P j | P b based on √(β i ) I E /R j and D/T ratio | |
| λ LT = uvλ √( β i ) |
When comparing the two methods, the main difference appears to be the method used to evaluate flexural strength (P b ).
For a detailed explanation of the steel beam construction method, see the article Practical example of constructing a steel beam .
Detect flexural and torsional buckling
Torsional buckling example
Data:
- Consider a simply supported beam with no intermediate restraints
- Support extension 6m
- Maximum design bending moment 100 kNm
As explained above, there are two methods to detect flexural and torsional buckling. Let's explain this with a practical example.
For a section to be resistant to bending, it must satisfy the following equation.
M X < M b /M LT
For simplicity, intermediate constraints are not considered in this example.
Then,
M LT = 0.925, Table 18, BS 5950
M b =P b S X Section 4.3.6.4
First, let's check the buckling resistance with a rigorous method.
The following route data is taken into account in the calculation
- T = 500mm
- T = 16mm
- t = 10mm
- B = 200mm
- b = 100mm
- R 1 = 20 mm
- d = 500 – 16 x 2 – 2 x 20 = 428 mm
- S X = 2175 × 10 3 mm 3
- Z X = 1914 × 10 3 mm 3
- R j = 43.3 mm
- The section is made of plastic according to its dimensions.
Rigorous method
Mb = PbSX
P b is a function of λ LT and P j
λ LT =uvλ√(β i )
λ = I E /R j
M E – can be obtained from Table 13 (Section 4.3.5.1) and takes into account L LT = L – span
Therefore,
M E = 1.0L LT = 1 x 6 = 6 meters
λ = I E /R j = 6,000/43.3 = 138.568
Section 4.3.6.8 applies to laminated profiles I and H
x = D / T used with u = 0.9
x = D / T = 500/16 = 31.25
β i can be found in Section 4.3.6.9
β i = 1 for class 1 plastic profiles or class 2 compact profiles
v – slenderness factor – is derived from Table 19 according to λ / x and η
λ/x = 138.568/31.25 = 4.434
For flanges of the same size η = 0.5
v = 0.84 from Table 19 ( conservative value assumed; λ/x = 4.5, interpolation should be used for exact value )
λ LT =uvλ√(β i ) = 0.9 x 0.84 x 138.568 x √(1) = 104.8
λ ELA can be found in Table 16 (indicated below in Table 16)
If λ ELA ≥λ LT ; P b =P j or else P b can be found in Table 16 for rolled profiles.
If λ ELA ≥λ LT It is not necessary to take torsional buckling into account; otherwise, a torsional buckling check must be carried out.
P j = 275 N/mm 2 ; λ ELA = 34.3
λ SHE <λ LT
Therefore, check for torsional buckling
From Table 16 for λ LT = 104.8; P b = 117 N/mm 2
M b =P b S X = 117 x 2175 x 10 3 x 10 -6 = 254.5 kN m
M b /M LT = 254.8 / 0.925 = 275.4 kNm
Therefore, MX = 100kNm
The cross section is suitable for lateral torsional buckling according to the strict method.
Simplified method
We do not need to do both calculations to check the buckling resistance.
M b =P b S X : Paragraph 4.3.7
The purpose of P b is not the same as the rigorous method.
This method provides conservative answers.
P b can be obtained from Table 20 of BS 5950 according to √(β i ) (EU E /R j ) and D/T
β i = 1; same as the previous calculation.
M E /R j = 138.568; from the above calculations
√(β i ) (I E /R j ) = 1 0.5 x 135.568 = 138.568
D/T = 500/16 = 31.25
Now you can get from table 20
P b = 116.646 N/mm 2
M b = P b S
M b /M LT = 253.705 / 0.925 = 274.3 kNm
So M is X = 100kNm
The section is therefore sufficient.